Judging from some of the feedback I got on my post on sterile neutrinos, it sounds like I lost some of my audience halfway through the post. I can't blame you. On the other hand, one blogger linked to my blog with the comment, "Caution: May cause stretch marks on your brain," which suggests that some of you enjoy the intellectual challenge. Striking the right balance can be difficult.
Looking back at what I wrote, I'm guessing that one of the speed bumps was the symmetry group for the standard model of particle physics, U(1) x SU(2) x SU(3), and the concept of symmetries generally. So I'm going to take another crack at explaining it.
In the world of particle physics, the bottom line is a quantity known as the Lagrangian density. This is a generalization of the Lagrangian of classical physics, which is simply the difference between the kinetic energy and the potential energy of the system being studied. For example, if you were studying a hypothetical solar system consisting of a single planet orbiting a star, the Lagrangian would be the sum of the kinetic energies of the planet and the star, minus the gravitational potential energy between them. If you know the Lagrangian, then you can figure out the equations of motion of the particles, through a tedious but straightforward mathematical procedure.
Heisenberg's Uncertainty Principle tells us that subatomic particles do not have well-defined paths. The Lagrangian is then replaced by a Lagrangian density, but the underlying principle is the same: If you know the Lagrangian density, you can figure out the probability that the particles will follow certain paths, through another elaborate but well-defined mathematical procedure.
Particle physics is the quest to find a Lagrangian density that explains everything we observe about the universe. But there is more to it than that. Particle physicists are strongly disposed to believe that this ultimate Lagrangian density, or Theory of Everything, must be simple and elegant. In fact, they expect the Theory of Everything to be so compelling in its simplicity and elegance that, when we finally see and understand it, we will exclaim: It could be no other way! Einstein was expressing a thought much like this when he said, "What really interests me is whether God had any choice in the creation of the world."
In this respect, the particle physicist's vision is much like the mathematician's. Just as all the theorems of plane geometry are derived from a few axioms, so the complexity and diversity of the universe is derived from a minimal Lagrangian density. This Lagrangian density should have as few free parameters as possible.
Particle physicists have come to view symmetries as hallmarks of simplicity and elegance in a Lagrangian density. A symmetry can be thought of as a rule of repetition. For example, an equilateral triangle is more symmetric than an ordinary triangle, because each of its sides and vertices is identical with the others, whereas the ordinary triangle has three different side lengths and vertex angles. A square is more symmetric still, because it has four identical side lengths and vertex angles. And so on, until you come to the limiting case of a circle, where every point on the circle is indistinguishable from every other. The circle is the most symmetric of all two-dimensional geometric figures, just as the sphere is the most symmetric of all geometric solids.
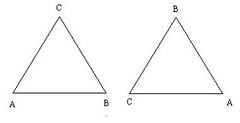
From the mathematical perspective, a more useful way of defining a particular kind of symmetry is by the ways the symmetric object can be rotated or flipped around so that its appearance is left unchanged. We'll illustrate how this works using a mathematical game.1 Suppose you center a drawing of an equilateral triangle on a round transparency. (The transparency must be round so that it will not provide any extra clues in the wager which follows.) Now suppose you place the transparency on a desktop, allow a friend to carefully note the orientation of the triangle, then wager your friend that he will not be able to tell whether you have moved the transparency if he steps out of the room for a minute. How can you win the wager?
It will be immediately obvious if you move the transparency to a different place on the desk. It will also be obvious if you rotate the transparency in place by any angle other than 120 or 240 degrees. But if you rotates the transparency in place by exactly 120 or 240 degrees, your friend will not be able to tell - unless he cheats by surreptitiously marking the transparency in some way, which breaks the symmetry.

An equilateral triangle before and after being rotated by 120 degrees. The triangles are distinguishable only because of the labels on the vertices, which break the symmetry.
You can also move the transparency in an undetectable way by flipping it over and returning it to its original position on the desktop. The trick is to flip it so that one vertex remains at the same position on the desk, while the other two swap places. There are three ways to do this, depending on which vertex you leave in place.
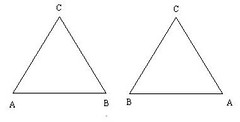
An equilateral triangle before and after being flipped. Again, the triangles can be distinguished only because of the labels on the vertices.
Now suppose you notice that your friend has surreptitiously marked one of the vertices. You can no longer leave him mystified by rotating the transparency, because the symmetry has been broken. However, if you flip the transparency so that the marked vertex is the one that remains in place, you can still win the wager. This shows that it is possible to break part of the symmetry of a geometric object without losing all the symmetry.
You can play the same game with a drawing of a square. In this case, you can rotate by 90, 180, or 270 degrees, or flip the diagram in four different ways, to win the wager. If you use a drawing of a rectangle, you can still rotate by 180 degrees or flip two different ways to win the wager. The most interesting case is a circle. You can rotate the circle by any angle at all or flip it around any axis at all and it remains unchanged. Symmetries of this kind, which work for any value of a continuous parameter (such as rotation angle), are a geometrical illustration of a mathematical concept called a Lie group. (Lie was the name of the Norwegian mathematician who invented this concept.) Don't let the algebra part scare you; I will try to keep the algebra to an absolute minimum.
The different things you can do to a symmetric object that leave it unchanged can be combined at will. For example, rotating an equilateral triangle by 240degrees is the same as rotating it twice by 120 degrees. Rotating it by 240 degrees and then by 120 degrees is the same as not rotating it at all - so the rotation by 240 degrees is the inverse of rotation by 120 degrees. Flipping the triangle on the same axis twice leaves the triangle unchanged, so each flip operation is its own inverse. In general, every operation permitted by a particular symmetry has an inverse operation, and any combination of two operations is identical to another single operation. This means that the operations permitted by a particular symmetry form the algebraic structure that mathematicians call a group. (That explains the other part of the name, "Lie group.") A very thorough discussion of the triangle symmetry group can be found here.
Mathematicians coin symbols for the various symmetry groups. For example, the symmetry group of the equilateral triangle is denoted D3. The symmetry groups for polyhedra in three dimensions use identical notation, and are of great interest to crystallographers and computational chemists. However, particle physicists are almost exclusively concerned with Lie groups, such as the symmetry group for the circle. The mathematical symbol for this symmetry group is U(1). So now at least you know what the first part of U(1) x SU(2) x SU(3) means, though I have not yet explained what it has to do with particle physics. Before we get to that, we need to talk about some Lie groups that are of interest to both classical and particle physics.
We'll do this with a thought experiment. Suppose, like Captain Kirk in James Blish's adaption of the Star Trek episode, "The Tholian Web," you found yourself floating in your own private universe, which is otherwise completely empty. What is your location, and which way is up? Good questions. You have no point of reference except for yourself, and nothing else to refer to. "Location" and "direction" have no meaning.
Now suppose Spock joins you in your heretofore private universe. What is his distance, and in what direction? Distance is certainly meaningful. He's thirty meters away. But direction is still not meaningful. Direction relative to what?
Now let McCoy join the two of you. Distance to McCoy is meaningful; he's forty meters away. Direction can be partially specified; the direction to McCoy is at right angles (90 degrees) to the direction to Spock. But that's still not a full specification of direction.
Mr. Scott now joins the party. You find that he is eighty meters away in a direction at right angles to both Spock and McCoy. You now have almost the full makings of a coordinate system. You can place the origin at your own location. The X-axis can be in the direction of Mr. Spock, the Y-axis can be in the direction of McCoy, and the Z-axis can be in the direction of Scotty. The only remaining wrinkle is that there are two directions at right angles to both Mr. Spock and McCoy, and you have to specify which is the direction to Scotty. Suppose that Mr. Spock is directly behind you, and McCoy directly beneath your feet. Scotty could be either directly to your right or directly to your left. If he's to your right, the coordinate system you've just set up is called a right-handed coordinate system. If he's to your left, then you have a left-handed coordinate system. Physicists normally prefer right-handed coordinate systems, for no particular good reason except that you have to choose one or the other. Mathematicians historically made the opposite choice, which has led to no end of confusion.
Now suppose, while you are all napping, the Tholians come along and move all of you exactly one hundred meters in Spock's direction. One hundred meters relative to what? In your private universe, position is measured relative to your own position. You can talk about moving everyone the same direction by the same distance, but it doesn't actually mean anything. You can't tell it happened. Position is a symmetry of your private universe. The group representing this symmetry is a Lie group, since the distance you all move can take any value from zero to infinity. This particular symmetry is called translational symmetry.
Now suppose the Tholians come along and, while you are once again napping, swap everyone around. Spock was originally thirty meters in the direction of the X axis, McCoy was forty meters along the Y axis, and Scotty was eighty meters along the Z axis. After the Tholians are done playing their pranks, Spock is thirty meters along the Z axis, McCoy is forty meters along the X axis, and Scotty is eighty meters along the Y axis. How will you know anything changed? You won't, because directions are all relative to your three friends. This is another symmetry of your private universe. In fact, this symmetry is a special case of rotational symmetry in three dimensions. Your positions have all rotated by 120 degrees around an axis pointing in the x=1, y=1, z=1 direction. You could do a rotation around this axis by any angle you like - or around any axis by any angle you like - without any discernible change to your universe. Because there is a continuous range of values for the rotation, rotational symmetry is represented by another Lie group. Mathematicians call this Lie group SO(3).
Next, the Tholians sneak by and put you all in suspended animation for a thousand years. How do you know? Your watches and clocks were all stopped for a thousand years, too. Time symmetry is another symmetry of your private universe. The interval of suspended animation can take any value from zero to infinity, so this is another Lie group.
Finally, suppose the Tholians set you all in motion, with a velocity of one hundred kilometers per second in Scotty's direction. Velocity relative to what? Boost symmetry is another symmetry of your private universe.
Now for the practical application. Our universe is a bit crowded compared with the private universe we have just been discussing. But if God moved every particle in the universe 100 meters in the direction of Polaris, how would we know what He had done? If God rotated the entire universe by seventeen degrees around an axis pointed towards Polaris, how would we know? And so on. All the symmetries we have just described are symmetries of the real universe.
As I've mentioned in earlier posts, every continuous symmetry in the laws of physics implies the conservation of some quantity (Noether's Theorem.) Translational symmetry means that the laws of physics are identical at all points in space, and it also implies the conservation of linear momentum. Rotational symmetry means that the laws of physics act the same in every direction, and it also implies the conservation of angular momentum. Time symmetry means that the laws of physics are eternally unchanging, and it also implies the conservation of energy.
Boost symmetry is what Einstein's Special Theory of Relativity is all about. However, Einstein's contribution was greater than this. Einstein's work showed that rotational symmetry and boost symmetry are simply special cases of a higher symmetry. The Lie group corresponding to this symmetry is called the special Lorentz group, and its mathematical symbol is SL(2,C). Furthermore, the Lorentz group and the translational and time symmetry groups can be combined into a single symmetry group, called the Poincare group.
There are two more symmetries in classical physics, but they are not Lie symmetries, since they do not depend on any continuous parameter. One is parity symmetry. Suppose, like Spock in James Blish's Spock Must Die!, you and all your friends are fed through a malicious Tholian transporter that reverses left and right. How would you know? With Spock directly behind you and McCoy directly below you, Scotty is now directly to your left rather than to your right - but the transporter has also reversed your brain, so you think right is left and vice versa. You cannot tell anything has happened.
Finally, suppose time runs backwards in your private universe. How would you know? Your brain and all your clocks run backwards also. This symmetry is called time reversal symmetry.
The symmetries just described - Poincare, parity, and time reversal - are the classical symmetries of physics. How these apply to particle physics, and the additional symmetries described by U(1) x SU(2) x SU(3), will be the topic of future posts.
1Ubergeeks like myself do this kind of thing all the time. This might explain our social lives. But it could be worse.